Logo ROOT   6.16/01
Reference Guide
rf201_composite.C File Reference
Tutorials » RooFit Tutorials

Detailed Description

View in nbviewer Open in SWAN 'ADDITION AND CONVOLUTION' RooFit tutorial macro #201

Composite p.d.f with signal and background component

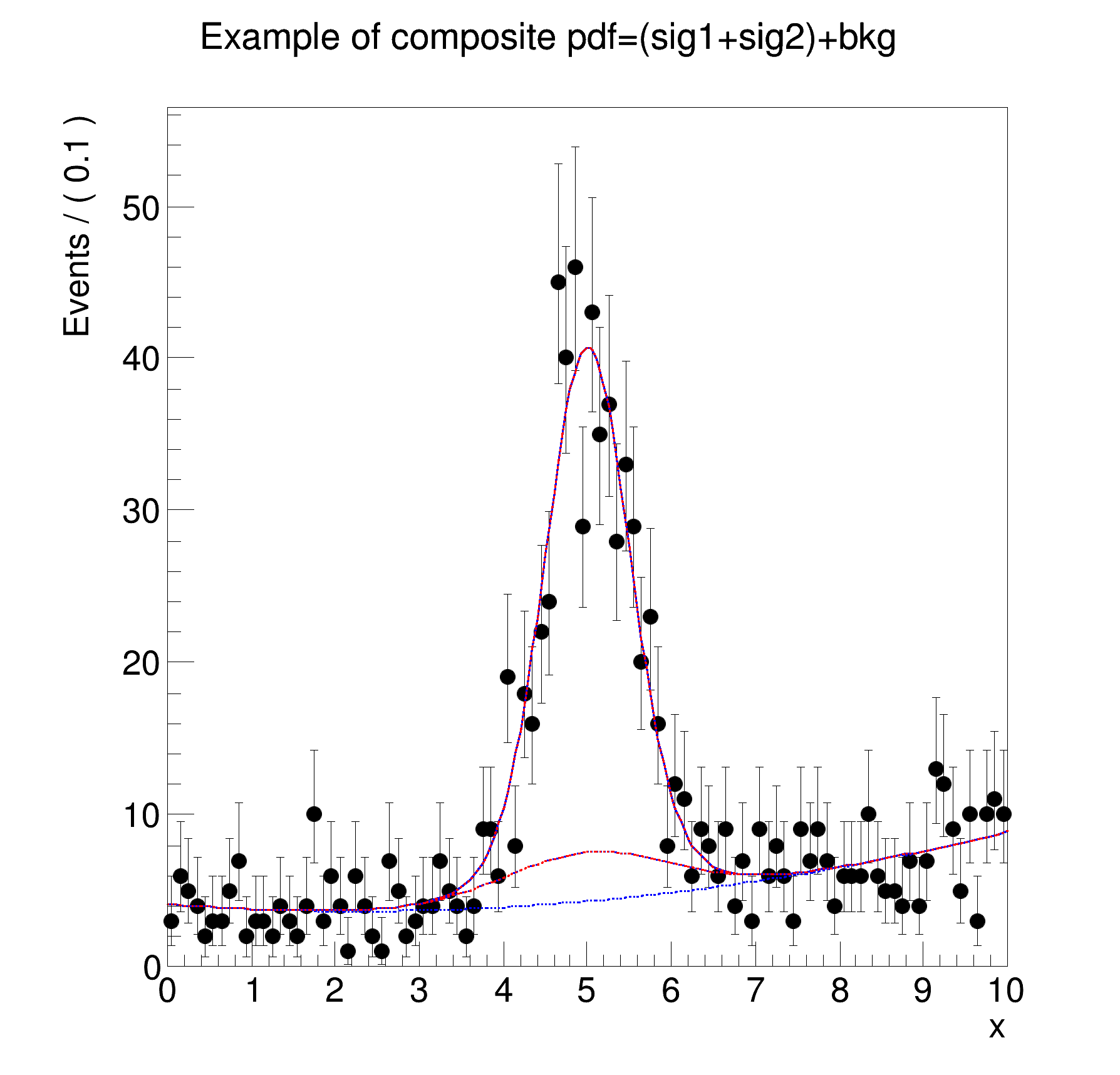
pdf = f_bkg * bkg(x,a0,a1) + (1-fbkg) * (f_sig1 * sig1(x,m,s1 + (1-f_sig1) * sig2(x,m,s2)))

␛[1mRooFit v3.60 -- Developed by Wouter Verkerke and David Kirkby␛[0m
Copyright (C) 2000-2013 NIKHEF, University of California & Stanford University
All rights reserved, please read http://roofit.sourceforge.net/license.txt
[#1] INFO:Minization -- RooMinimizer::optimizeConst: activating const optimization
[#1] INFO:Minization -- The following expressions have been identified as constant and will be precalculated and cached: (sig1,sig2)
[#1] INFO:Minization -- The following expressions will be evaluated in cache-and-track mode: (bkg)
**********
** 1 **SET PRINT 1
**********
**********
** 2 **SET NOGRAD
**********
PARAMETER DEFINITIONS:
NO. NAME VALUE STEP SIZE LIMITS
1 a0 5.00000e-01 1.00000e-01 0.00000e+00 1.00000e+00
2 a1 2.00000e-01 1.00000e-01 0.00000e+00 1.00000e+00
3 bkgfrac 5.00000e-01 1.00000e-01 0.00000e+00 1.00000e+00
4 sig1frac 8.00000e-01 1.00000e-01 0.00000e+00 1.00000e+00
**********
** 3 **SET ERR 0.5
**********
**********
** 4 **SET PRINT 1
**********
**********
** 5 **SET STR 1
**********
NOW USING STRATEGY 1: TRY TO BALANCE SPEED AGAINST RELIABILITY
**********
** 6 **MIGRAD 2000 1
**********
FIRST CALL TO USER FUNCTION AT NEW START POINT, WITH IFLAG=4.
START MIGRAD MINIMIZATION. STRATEGY 1. CONVERGENCE WHEN EDM .LT. 1.00e-03
FCN=1962.68 FROM MIGRAD STATUS=INITIATE 10 CALLS 11 TOTAL
EDM= unknown STRATEGY= 1 NO ERROR MATRIX
EXT PARAMETER CURRENT GUESS STEP FIRST
NO. NAME VALUE ERROR SIZE DERIVATIVE
1 a0 5.00000e-01 1.00000e-01 2.01358e-01 5.55984e+00
2 a1 2.00000e-01 1.00000e-01 2.57889e-01 -1.57464e+00
3 bkgfrac 5.00000e-01 1.00000e-01 2.01358e-01 1.16417e+00
4 sig1frac 8.00000e-01 1.00000e-01 2.57889e-01 -2.02114e+00
ERR DEF= 0.5
MIGRAD MINIMIZATION HAS CONVERGED.
MIGRAD WILL VERIFY CONVERGENCE AND ERROR MATRIX.
COVARIANCE MATRIX CALCULATED SUCCESSFULLY
FCN=1962.27 FROM MIGRAD STATUS=CONVERGED 77 CALLS 78 TOTAL
EDM=2.21873e-05 STRATEGY= 1 ERROR MATRIX ACCURATE
EXT PARAMETER STEP FIRST
NO. NAME VALUE ERROR SIZE DERIVATIVE
1 a0 4.41621e-01 7.31971e-02 4.49448e-03 -2.95364e-02
2 a1 2.01070e-01 1.18164e-01 5.76018e-03 4.48544e-03
3 bkgfrac 5.04184e-01 3.60469e-02 1.24368e-03 -2.15278e-02
4 sig1frac 8.37334e-01 1.17186e-01 6.00443e-03 1.16304e-03
ERR DEF= 0.5
EXTERNAL ERROR MATRIX. NDIM= 25 NPAR= 4 ERR DEF=0.5
5.397e-03 1.212e-03 -3.088e-04 -1.017e-03
1.212e-03 1.439e-02 -3.249e-03 -9.741e-03
-3.088e-04 -3.249e-03 1.302e-03 3.287e-03
-1.017e-03 -9.741e-03 3.287e-03 1.422e-02
PARAMETER CORRELATION COEFFICIENTS
NO. GLOBAL 1 2 3 4
1 0.14102 1.000 0.138 -0.117 -0.116
2 0.77022 0.138 1.000 -0.751 -0.681
3 0.82636 -0.117 -0.751 1.000 0.764
4 0.78133 -0.116 -0.681 0.764 1.000
**********
** 7 **SET ERR 0.5
**********
**********
** 8 **SET PRINT 1
**********
**********
** 9 **HESSE 2000
**********
COVARIANCE MATRIX CALCULATED SUCCESSFULLY
FCN=1962.27 FROM HESSE STATUS=OK 23 CALLS 101 TOTAL
EDM=2.21633e-05 STRATEGY= 1 ERROR MATRIX ACCURATE
EXT PARAMETER INTERNAL INTERNAL
NO. NAME VALUE ERROR STEP SIZE VALUE
1 a0 4.41621e-01 7.31875e-02 8.98897e-04 -1.17025e-01
2 a1 2.01070e-01 1.17637e-01 2.30407e-04 -6.40829e-01
3 bkgfrac 5.04184e-01 3.59091e-02 2.48735e-04 8.36870e-03
4 sig1frac 8.37334e-01 1.16852e-01 2.40177e-04 7.40515e-01
ERR DEF= 0.5
EXTERNAL ERROR MATRIX. NDIM= 25 NPAR= 4 ERR DEF=0.5
5.396e-03 1.199e-03 -3.049e-04 -1.005e-03
1.199e-03 1.426e-02 -3.211e-03 -9.629e-03
-3.049e-04 -3.211e-03 1.292e-03 3.259e-03
-1.005e-03 -9.629e-03 3.259e-03 1.414e-02
PARAMETER CORRELATION COEFFICIENTS
NO. GLOBAL 1 2 3 4
1 0.14012 1.000 0.137 -0.116 -0.115
2 0.76777 0.137 1.000 -0.748 -0.678
3 0.82488 -0.116 -0.748 1.000 0.763
4 0.77985 -0.115 -0.678 0.763 1.000
[#1] INFO:Minization -- RooMinimizer::optimizeConst: deactivating const optimization
[#1] INFO:Plotting -- RooAbsPdf::plotOn(model) directly selected PDF components: (bkg)
[#1] INFO:Plotting -- RooAbsPdf::plotOn(model) indirectly selected PDF components: ()
[#1] INFO:Plotting -- RooAbsPdf::plotOn(model) directly selected PDF components: (bkg,sig2)
[#1] INFO:Plotting -- RooAbsPdf::plotOn(model) indirectly selected PDF components: (sig)
0x7fff063318b8 RooAddPdf::model = 0.898624 [Auto,Dirty]
0x7fff06333308/V- RooChebychev::bkg = 0.79893 [Auto,Dirty]
0x7fff06335820/V- RooRealVar::x = 5
0x7fff06333ca0/V- RooRealVar::a0 = 0.441621 +/- 0.0731875
0x7fff06333880/V- RooRealVar::a1 = 0.20107 +/- 0.117637
0x7fff06332070/V- RooRealVar::bkgfrac = 0.504184 +/- 0.0359091
0x7fff063325e0/V- RooAddPdf::sig = 1 [Auto,Dirty]
0x7fff06334638/V- RooGaussian::sig1 = 1 [Auto,Dirty]
0x7fff06335820/V- RooRealVar::x = 5
0x7fff06335400/V- RooRealVar::mean = 5
0x7fff06334fd0/V- RooRealVar::sigma1 = 0.5
0x7fff06332d98/V- RooRealVar::sig1frac = 0.837334 +/- 0.116852
0x7fff063340c0/V- RooGaussian::sig2 = 1 [Auto,Dirty]
0x7fff06335820/V- RooRealVar::x = 5
0x7fff06335400/V- RooRealVar::mean = 5
0x7fff06334bb0/V- RooRealVar::sigma2 = 1
[#1] INFO:Plotting -- RooAbsPdf::plotOn(model) directly selected PDF components: (bkg,sig2)
[#1] INFO:Plotting -- RooAbsPdf::plotOn(model) indirectly selected PDF components: ()
0x7fff06330790 RooAddPdf::model = 0.898624 [Auto,Dirty]
0x7fff06333308/V- RooChebychev::bkg = 0.79893 [Auto,Dirty]
0x7fff06335820/V- RooRealVar::x = 5
0x7fff06333ca0/V- RooRealVar::a0 = 0.441621 +/- 0.0731875
0x7fff06333880/V- RooRealVar::a1 = 0.20107 +/- 0.117637
0x7fff06332070/V- RooRealVar::bkgfrac = 0.504184 +/- 0.0359091
0x7fff06334638/V- RooGaussian::sig1 = 1 [Auto,Dirty]
0x7fff06335820/V- RooRealVar::x = 5
0x7fff06335400/V- RooRealVar::mean = 5
0x7fff06334fd0/V- RooRealVar::sigma1 = 0.5
0x55b14014b880/V- RooRecursiveFraction::model_recursive_fraction_sig1 = 0.415163 [Auto,Clean]
0x7fff06332d98/V- RooRealVar::sig1frac = 0.837334 +/- 0.116852
0x7fff06332070/V- RooRealVar::bkgfrac = 0.504184 +/- 0.0359091
0x7fff063340c0/V- RooGaussian::sig2 = 1 [Auto,Dirty]
0x7fff06335820/V- RooRealVar::x = 5
0x7fff06335400/V- RooRealVar::mean = 5
0x7fff06334bb0/V- RooRealVar::sigma2 = 1
0x55b1404b2b50/V- RooRecursiveFraction::model_recursive_fraction_sig2 = 0.0806524 [Auto,Clean]
0x55b1404b7e80/V- RooConstVar::1 = 1
0x7fff06332d98/V- RooRealVar::sig1frac = 0.837334 +/- 0.116852
0x7fff06332070/V- RooRealVar::bkgfrac = 0.504184 +/- 0.0359091
#include "RooRealVar.h"
#include "RooDataSet.h"
#include "RooGaussian.h"
#include "RooChebychev.h"
#include "RooAddPdf.h"
#include "TCanvas.h"
#include "TAxis.h"
#include "RooPlot.h"
using namespace RooFit ;
void rf201_composite()
{
// S e t u p c o m p o n e n t p d f s
// ---------------------------------------
// Declare observable x
RooRealVar x("x","x",0,10) ;
// Create two Gaussian PDFs g1(x,mean1,sigma) anf g2(x,mean2,sigma) and their parameters
RooRealVar mean("mean","mean of gaussians",5) ;
RooRealVar sigma1("sigma1","width of gaussians",0.5) ;
RooRealVar sigma2("sigma2","width of gaussians",1) ;
RooGaussian sig1("sig1","Signal component 1",x,mean,sigma1) ;
RooGaussian sig2("sig2","Signal component 2",x,mean,sigma2) ;
// Build Chebychev polynomial p.d.f.
RooRealVar a0("a0","a0",0.5,0.,1.) ;
RooRealVar a1("a1","a1",0.2,0.,1.) ;
RooChebychev bkg("bkg","Background",x,RooArgSet(a0,a1)) ;
// ---------------------------------------------
// M E T H O D 1 - T w o R o o A d d P d f s
// =============================================
// A d d s i g n a l c o m p o n e n t s
// ------------------------------------------
// Sum the signal components into a composite signal p.d.f.
RooRealVar sig1frac("sig1frac","fraction of component 1 in signal",0.8,0.,1.) ;
RooAddPdf sig("sig","Signal",RooArgList(sig1,sig2),sig1frac) ;
// A d d s i g n a l a n d b a c k g r o u n d
// ------------------------------------------------
// Sum the composite signal and background
RooRealVar bkgfrac("bkgfrac","fraction of background",0.5,0.,1.) ;
RooAddPdf model("model","g1+g2+a",RooArgList(bkg,sig),bkgfrac) ;
// S a m p l e , f i t a n d p l o t m o d e l
// ---------------------------------------------------
// Generate a data sample of 1000 events in x from model
RooDataSet *data = model.generate(x,1000) ;
// Fit model to data
model.fitTo(*data) ;
// Plot data and PDF overlaid
RooPlot* xframe = x.frame(Title("Example of composite pdf=(sig1+sig2)+bkg")) ;
data->plotOn(xframe) ;
model.plotOn(xframe) ;
// Overlay the background component of model with a dashed line
model.plotOn(xframe,Components(bkg),LineStyle(kDashed)) ;
// Overlay the background+sig2 components of model with a dotted line
model.plotOn(xframe,Components(RooArgSet(bkg,sig2)),LineStyle(kDotted)) ;
// Print structure of composite p.d.f.
model.Print("t") ;
// ---------------------------------------------------------------------------------------------
// M E T H O D 2 - O n e R o o A d d P d f w i t h r e c u r s i v e f r a c t i o n s
// =============================================================================================
// Construct sum of models on one go using recursive fraction interpretations
//
// model2 = bkg + (sig1 + sig2)
//
RooAddPdf model2("model","g1+g2+a",RooArgList(bkg,sig1,sig2),RooArgList(bkgfrac,sig1frac),kTRUE) ;
// NB: Each coefficient is interpreted as the fraction of the
// left-hand component of the i-th recursive sum, i.e.
//
// sum4 = A + ( B + ( C + D) with fraction fA, fB and fC expands to
//
// sum4 = fA*A + (1-fA)*(fB*B + (1-fB)*(fC*C + (1-fC)*D))
// P l o t r e c u r s i v e a d d i t i o n m o d e l
// ---------------------------------------------------------
model2.plotOn(xframe,LineColor(kRed),LineStyle(kDashed)) ;
model2.plotOn(xframe,Components(RooArgSet(bkg,sig2)),LineColor(kRed),LineStyle(kDashed)) ;
model2.Print("t") ;
// Draw the frame on the canvas
new TCanvas("rf201_composite","rf201_composite",600,600) ;
gPad->SetLeftMargin(0.15) ; xframe->GetYaxis()->SetTitleOffset(1.4) ; xframe->Draw() ;
}
kTRUE
const Bool_t kTRUE
Definition: RtypesCore.h:87
kRed
@ kRed
Definition: Rtypes.h:63
kDashed
@ kDashed
Definition: TAttLine.h:48
kDotted
@ kDotted
Definition: TAttLine.h:48
gPad
#define gPad
Definition: TVirtualPad.h:286
RooAddPdf
RooAddPdf is an efficient implementation of a sum of PDFs of the form.
Definition: RooAddPdf.h:29
RooArgSet
RooArgSet is a container object that can hold multiple RooAbsArg objects.
Definition: RooArgSet.h:28
RooChebychev
Chebychev polynomial p.d.f.
Definition: RooChebychev.h:25
RooDataSet
RooDataSet is a container class to hold unbinned data.
Definition: RooDataSet.h:31
RooGaussian
Plain Gaussian p.d.f.
Definition: RooGaussian.h:25
RooPlot
A RooPlot is a plot frame and a container for graphics objects within that frame.
Definition: RooPlot.h:41
RooPlot::GetYaxis
TAxis * GetYaxis() const
Definition: RooPlot.cxx:1123
RooPlot::Draw
virtual void Draw(Option_t *options=0)
Draw this plot and all of the elements it contains.
Definition: RooPlot.cxx:558
RooRealVar
RooRealVar represents a fundamental (non-derived) real valued object.
Definition: RooRealVar.h:36
TAttAxis::SetTitleOffset
virtual void SetTitleOffset(Float_t offset=1)
Set distance between the axis and the axis title Offset is a correction factor with respect to the "s...
Definition: TAttAxis.cxx:294
TCanvas
The Canvas class.
Definition: TCanvas.h:31
x
Double_t x[n]
Definition: legend1.C:17
RooFit::Components
RooCmdArg Components(const RooArgSet &compSet)
Definition: RooGlobalFunc.cxx:73
RooFit::LineColor
RooCmdArg LineColor(Color_t color)
Definition: RooGlobalFunc.cxx:55
RooFit::LineStyle
RooCmdArg LineStyle(Style_t style)
Definition: RooGlobalFunc.cxx:56
rf201_composite
Definition: rf201_composite.py:1
xmlio::Title
const char * Title
Definition: TXMLSetup.cxx:67
Author
07/2008 - Wouter Verkerke

Definition in file rf201_composite.C.